Experimental verification of dual nature of matter-wave or Davisson – Germer
Experiment
Fig (a)
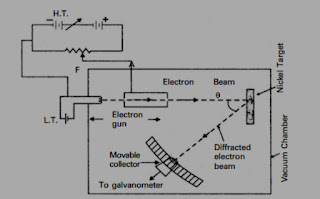
The experimental arrangement of the Davisson and Germer experiment is shown in fig.
The electrons are emitted from a heated filament and are accelerated through a variable potential
‘V’ and emerge from the electron gun. This electron beam incident normally on a nickel crystal
and get diffracted from the crystal in all directions. These diffracted electrons are collected by the
movable collector. And a galvanometer is connected to it to measure the current. If more
electrons are collected by the collector then there will be more deflection is observed in the
galvanometer vice versa. When voltage ‘V’ is given to a lower value and the crystal is turned to
any arbitrary azimuth. The collected is moved to various positions and the galvanometer current
is noted. The current is a measure of the intensity of the diffracted beam is plotted against the angle
φ. The observations are repeated for different accelerating potentials and the corresponding
curves are obtained as shown below.
From the graphs (Fig b), it can be concluded that a ‘bump’ begins to appear in the curve
for 44 V. With increasing potential, the bump moves upwards and becomes most prominent in the
the curve for 54 V and φ =50o
at higher potentials the bump gradually disappears, the bump in its
most prominent state verifies the existence of electron waves.
According to de Broglie, the wavelength associated with an electron accelerated through ‘V’
volts is
𝜆 =12.27/√𝑉 𝐴`0
V = 54 V then λ = 1.67 𝐴 (1)
In the case of nickel crystal, the grating space = d = 0.91 𝐴
From the Davisson and Germer experiment, θ =180−50/2= 65𝑜
From Bragg’s law (x-ray diffraction),
2d sinθ = nλ
n=1, d = 0.91 𝐴
, θ = 65𝑜
2X0.91Xsin 65𝑜= λ
λ = 1.65 𝐴 (2)
compare eq.(1) and eq.(2), it can be concluded that, the electron is showing dual nature of matter waves.